Quadrilaterals

Theorem 1 -Angle sum property of Quadrilateral:

The sum of four angles of a Quadrilateral is $\text{360°}$

 

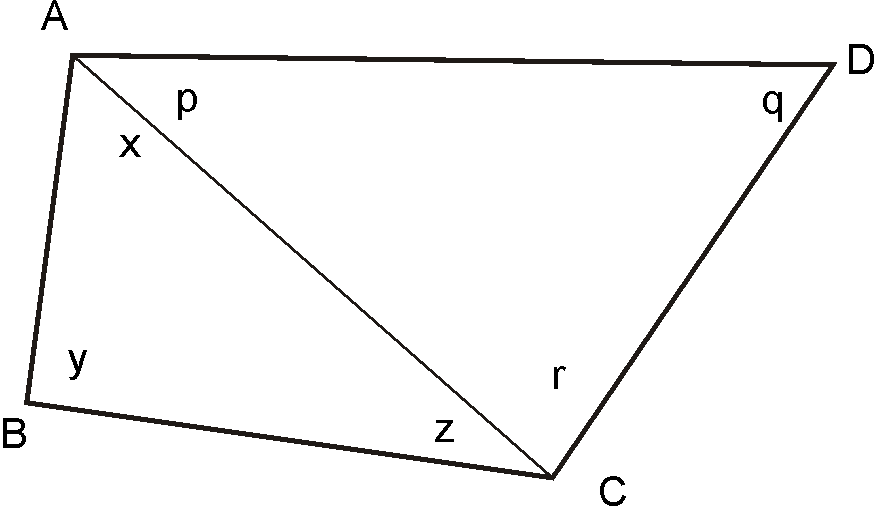
Given: A Quadrilateral $\text{ABCD}$.

To prove :

$\angle \text{A + }\angle \text{B + }\angle \text{C + }\angle \text{D = 360°}$

Construction: Join AC

Proof: In $\Delta \text{ABC}$

$\text{x + y + z = 180°}$ (By angle sum property of triangle)

In $\Delta \text{ADC}$

$\text{p + q + r = 180°}$ (By angle sum property of triangle)

So, adding

$\text{x + y + z + p + q + r = 180° + 180°}$

$\left(\text{x + p}\right)\text{ + y + }\left(\text{z + r}\right)\text{ + q = 360°}$

$\angle \text{A + }\angle \text{B + }\angle \text{C + }\angle \text{D = 360°}$

 

Remarks:

·            A parallelogram is a trapezium. But a trapezium is not parallelogram.

·            A rectangle is parallelogram, But a parallelogram is not a rectangle.

·            A rhombus is parallelogram, but parallelogram is not a rhombus.

·            A square is parallelogram, rectangle, rhombus but not viceversa.

·            A kite is not a parallelogram.

 

Properties of parallelogram:

A Quadrilateral is parallelogram if both the pairs of opposite sides are parallel.

 

 

Theorem 2:

A diagonal of a parallelogram divides it into two congruent triangles.

Given:

$\text{ABCD}$ is parallelogram. $\text{AC}$ is diagonal

To prove:

$\Delta \text{ABC}\cong \Delta \text{ADC}$

Proof:

$\text{AD}\parallel \text{BC}$

And $\text{AC}$ is transversal

So $\angle \text{DAC = }\angle \text{ACB}$ …..(Alternate angles)

$\text{AB}\parallel \text{DC}$ and $\text{AC}$ is transversal

$\angle \text{BAC = }\angle \text{ACD}$ ……(Alternate angles)

$\text{AC = AC}$ …(Common)

$\Delta \text{ABC}\cong \Delta \text{CDA}$ …( By ASA rule.)

 

Theorem 3:

In a parallelogram, opposite sides are equal and opposite angles are equal.

Given :

$\text{ABCD}$ is parallelogram

To prove:

$\text{AB = CD}$

$\text{AD = BC}$

$\angle \text{B = }\angle \text{D}$ and $\angle \text{A = }\angle \text{C}$

Construction: Join AC

Proof:

$\text{AD}\parallel \text{BC}$

And $\text{AC}$ is transversal

$\angle \text{DAC = }\angle \text{ACB}$ …..(Alternate angles)

$\text{AB}\parallel \text{DC}$ and AC is transversal

$\angle \text{BAC = }\angle \text{ACD}$ ……(Alternate angles)

$\text{AC = AC}$ …(Common)

$\Delta \text{ABC}\cong \Delta \text{CDA}$ (By ASA rule.)

$\text{AB = CD}$

$\text{AD = BC}$

$\angle \text{B = }\angle \text{D}$ (CPCT).

Similarly it can be proved that $\angle \text{A = }\angle \text{C}$

 

Theorem 4:

The diagonals of a parallelogrambisect each other.

Given: $\text{ABCD}$ is a parallelogram

$\text{AB}\parallel \text{CD}$

Ac and $\text{BD}$ intersect at $\text{O}$.

To prove:

$\text{O}$ is midpoint of $\text{AC}$ and BD.

That is $\text{AO = OC, OB = OD}$

Proof:

In $\Delta \text{AOB}$ and $\Delta \text{DOC}$

$\angle OBA\text{ = }\angle ODC$ ….( Alternate angles, $\text{AB}\parallel \text{CD}$,BD as transversal)

$\angle OAB\text{ = }\angle OCD$ …. ( Alternate angles, $\text{AB}\parallel \text{CD}$,AC as transversal)

$\text{AB = CD}$ …… (Opp sides of parallelogram)

$\Delta \text{OBA}\cong \Delta \text{ODC}$ …..(By ASA rule.)

So $\text{OB = OD, AO = OC}$ (CPCT)

 

 

 

Theorem 5:

A Quadrilateral is parallelograms if opposite sides are congruent.

Given:

$\text{ABCD}$ is Quadrilateral

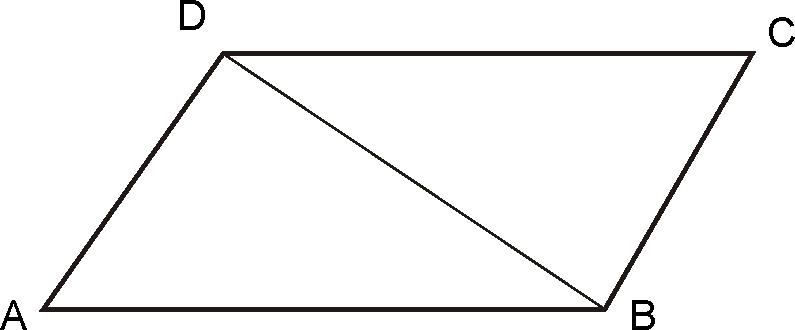
With $\text{AB = CD}$ and $\text{AD = BC}$

To prove: ABCD is parallelogram

i.e.

$\text{AB}\parallel \text{CD}$

$\text{AD}\parallel \text{BC}$

Construction:Join $\text{BD}$

Proof:

In $\Delta \text{ABD}$ and $\Delta \text{CDB}$

$\text{ AB = CD}$ ….(Given)

$\text{AD = BC}$ ….(Given)

$\text{BD = BD}$ …(Common)

$\Delta \text{ABD}\cong \Delta \text{CDB}$ ….(SSS rule)

$\angle \text{ABD = }\angle \text{CDB}$ ….. (CPCT)

$\text{AB}\parallel \text{CD}$ …..(Alternate angle test)

Similarly

$\angle \text{ADB = }\angle \text{DBC}$ (CPCT)

$\text{AD}\parallel \text{BC}$ …(Alternate angle test)

AS both the pair of opposite sides areparallel, we say $\text{ABCD}$ is parallelogram.

 

 

Theorem 6:

A Quadrilateral is parallelogram if opposite angles are congruent.

Given:

$\text{PQRS}$ is Quadrilateral

With $\angle \text{P = }\angle \text{R}$ and $\angle \text{S = }\angle \text{Q}$

To prove: $\text{PQRS}$ is parallelogram

i.e.

$\text{PQ}\parallel \text{RS}$

$\text{PS}\parallel \text{QR}$

Construction : Join $\text{PR}$

Proof:

$\angle \text{P = }\angle \text{R}$

$\angle \text{S = }\angle \text{Q}$

So $\angle \text{P + }\angle \text{S = }\angle \text{Q + }\angle \text{R}$ …(1)

But we know

$\angle \text{P + }\angle \text{S + }\angle \text{Q + }\angle \text{R = 360°}$ (Angle sum property of quadrilateral)…2

$\angle \text{Q + }\angle \text{R + }\angle \text{Q + }\angle \text{R = 360°}$ (From $\text{1}$ and $\text{2}$ )

$\therefore \text{2}\angle \text{Q + 2}\angle \text{R = 360°}$

$\angle \text{Q + }\angle \text{R = 180°}$

$\therefore \text{PQ}\parallel \text{RS}$ ( Sum of interior angle criteria)

Similarly,

$\text{PS}\parallel \text{QR}$ can be proved.

As both the pair of sides are parallel, we say $\text{PQRS}$ is parallelogram.

 

Theorem 7:

A Quadrilateral is parallelogram if a pair of opposite sides is congruent and parallel.

Given: $\text{ABCD}$ is quadrilateral.

$\text{AB = CD}$

$\text{AB}\parallel \text{CD}$

To prove: ABCD is parallelogram.

i.e. $\text{BC}\parallel \text{AD}$

Proof:

 

Construction: join AC

Proof:

In $\Delta \text{ADC}$ and $\Delta \text{CBA}$

$\text{AB = CD}$ …(Given)

$\angle \text{ACD = }\angle \text{BAC}$ …(Alternate angle as $\text{AB}\parallel \text{CD}$ )

$\text{AC = AC}$ …..(Common)

$\Delta \text{ADC}\cong \Delta \text{CBA}$ … (SAS rule)

So $\angle \text{DAC = }\angle \text{ACD}$ ….( CPCT)

$\text{BC}\parallel \text{AD}$ (Alternate angle criteria)

Converse :

 

 

 

Theorem 8 : If diagonals of parallelogram are equal then it is rectangle.

Given:

$\text{XYZW}$ is parallelogram

$\text{XZ, YW}$ be its diagonal.

$\text{XZ = YW}$

To prove: $\text{XYZW}$ is rectangle

i.e. $\angle \text{X = }\angle \text{Y = }\angle \text{Z = }\angle \text{W = 90°}$

Proof:

In $\Delta \text{XWZ}$ and $\Delta \text{YZW}$

$\text{XZ = YW}$ ….(Given)

$\text{XW = YZ}$ ….(Opp. side of parallelogram)

$\text{WZ =WZ}$ …(Common)

$\Delta \text{XWZ}\cong \Delta \text{YZW}$ …(SSS rule)

$\angle \text{W = }\angle \text{Z}$ (CPCT)…..1

But $\angle \text{W + }\angle \text{Z = 180}$ …(Interior angles are supplementary)

$\angle \text{W + }\angle \text{W = 180}$ …(From 1)

$\therefore \text{2}\angle \text{W = 180}$

$\therefore \angle \text{W = 90}$

Similarly we can show all the angles of quadrilateral are $\text{90°}$

Hence it is rectangle.

 

 

Theorem 9:

Diagonals of a rhombus are perpendicular to each other.

Given :

$\text{ABCD}$ is rhombus.

$\therefore \text{ABCD}$ is parallelogram.

$\text{AB = BC = CD = AD}$

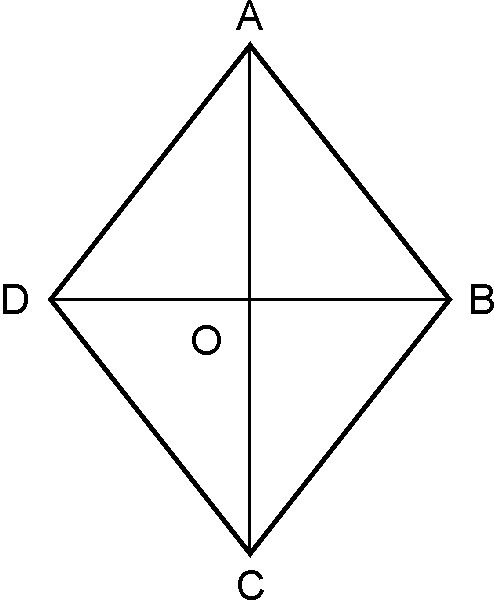
To prove:

$\text{AC}\perp \text{BD}$

$\angle \text{AOB = 90}$

Proof:

In $\Delta \text{AOB}$ and $\Delta \text{BOC}$

$\text{AB = BC}$ … .(Given)

$\text{AO = OC}$ ……. (Diagonals of parallelogram bisect each other)

$\text{OB = OB}$ ……..( Common)

$\Delta \text{AOB}\cong \Delta \text{COB}$ …(SSS test)

So $\angle \text{AOB = }\angle \text{BOC}$ …(CPCT)

But $\angle \text{AOB + }\angle \text{BOC = 180}°$ ….(Linear pair)

$\angle \text{AOB + }\angle \text{AOB = 180}°$

$\therefore 2\angle \text{AOB = 180}°$

$\therefore \angle \text{AOB = 90}°$

$\therefore \text{AC}\perp \text{BD}$

 

 

 

Theorem 10- Mid-point theorem:

The line segment joining the midpoint of any two sides of a triangle is parallel to third side and is half of third side.

Given: In $\Delta \text{ABC}$ $\text{D}$ and $\text{E}$ are midpoints of the sides $\text{AB}$ and $\text{AC}$ respectively.

To prove:

1) $\text{DE}\parallel \text{BC}$ 2) $\text{DE = }\frac{1}{2}\text{BC}$.

Construction: produce DE so that DE=EF. Join CF

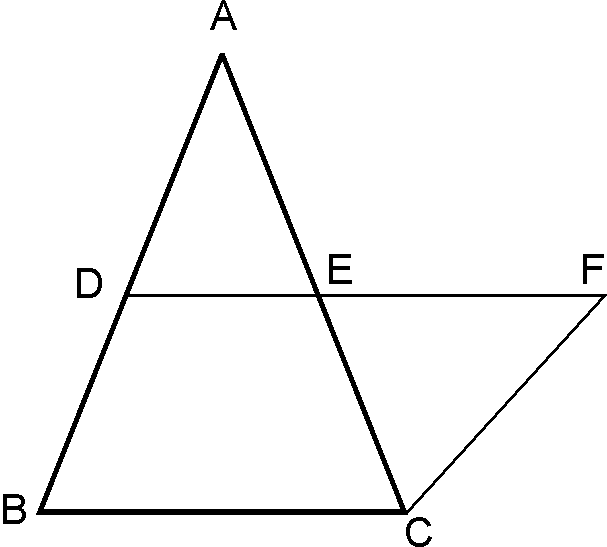
Proof:

In $\Delta \text{ADE}$ and $\Delta \text{CEF}$

$\text{AE = EC}$ …. (E is midpoint of AC)

$\text{DE = EF}$ ….. (By construction)

$\angle AED\text{ = }\angle CEF$ ……(V. opp angles)

$\Delta \text{ADE}\cong \Delta \text{CEF}$ …… (SAS rule)

$\angle \text{ADE = }\angle \text{EFC}$ …(CPCT)

$\text{AD = CF}$ ……. (CPCT)

But $\text{AD = BD}$ …….(D is midpoint)

So $\text{BD = CF}$

$\angle \text{ADE = }\angle \text{EFC}$ …(CPCT)

$\text{AD}\parallel \text{FC}$

$\square \text{DBCF}$ is parallelogram…… (One pair of side is parallel and equal)

So, $\text{DF}\parallel \text{BC}$

$\text{DF = BC}$

$\text{2 DE = BC}$ (By construction)

$\text{DE = }\frac{\text{1}}{\text{2}}\text{BC}$

 

 

 

Theorem 11 -Converse of midpoint theorem:

The line through the midpoint of one side of a triangle, parallel to other side, intersects the third side at its midpoint.

Given:

In $\Delta \text{ABC}$, $\text{D}$ is mid-point of $\text{AB}$

$\text{DE}\parallel \text{BC}$

To prove:

$\text{E}$ is midpoint of $\text{AC}$.

Proof:

Suppose $\text{E}$ is not midpoint of $\text{AC}$.

Let $\text{E}$ ’ is midpoint of $\text{AC}$.

Then By mid-point theorem, $\text{DE'}\parallel \text{BC}$

But $\text{DE}\parallel \text{BC}$ …….. (Given)

$\text{DE}$ and $\text{DE}$ ’ are two intersecting line are parallel to $\text{BC}$.

Contradicts the parallel line axiom.

So $\text{E}$ is mid-point of $\text{AC}$.