Introduction to Euclid’s Geometry

Euclid’s Definitions:

1) Point: A point is that which has no part.

2) Line: A line is breadthless length.

3) The ends of a line are points.

4) A straight line is one which lies evenly with the points on itself.

5) Surface: A surface is that which has length and breadth only.

6) The edges of surface are lines.

7) A plane surface is a surface which lies evenly with the straight lines on itself.

Axioms: Axioms are assumptions used throughout mathematics.

Some of Euclid’s axiom’s:

1) Things which are equal to the same thing are equal to one another.

2) If equals are added to equals, the wholes are equal.

3) If equals are subtracted from equals, the remainders are equal.

4) Things which coincide with one another are equal to one another.

5) The whole is greater than the part.

6) Things which are double of the same things are equal to one another.

7) Things which are halves of the same things are equal to one another.

Postulates: Postulates are the assumptions used specially for geometry.

Euclid’s five postulates.

Postulate 1: A straight line may be drawn from any one point to any other point.

Axiom: Given two points there can be one and only one line passing through them.

Postulate 2: A terminated line can be extended infinitely.

Axiom: A segment can be extended from both ends to form a line.

Postulate 3: A circle can be drawn of any radius and with any centre.

Postulate 4: All right angles are equal to one another.

Postulate 5: If a straight line falling on two straight lines makes the interior angles on the same side of it taken together less than two right angles, then the two straight lines, if produced indefinitely, meet on that side on which the sum of angles is less than two right angles.

OR

Two intersecting lines cannot be parallel to the same time.

OR

For every line l and point P not on line, there is a unique line passing through P and parallel to line l.

Theorem or preposition:

The result proved using axioms and postulates is called theorem or preposition.

Theorem 1:

Two distinct lines cannot have more than one point in common.

Given: Let $\text{l}$ and m be two lines.

To prove: $\text{l}$ and m have only one point in common.

Proof: Suppose two lines intersect in two distinct points P and Q.

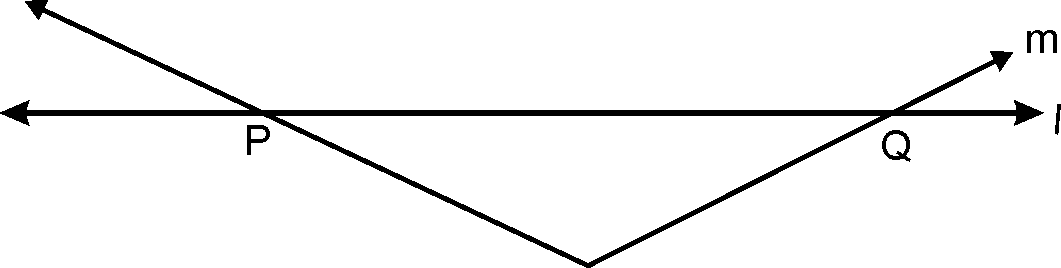
So we have two lines l and m passing through points P and Q..

But our assumption clashes with the axioms that only one line can pass through two distinct points.

So, our assumption is wrong.

So we conclude that two distinct lines cannot have more than one point in common.

Theorem2 - Two lines which are both parallel to the same line, are parallel to each other. 

Given: Three lines l, m, n in a plane such that m || l and n || l. 

To prove: m || n

Proof: If possible, let m be not parallel to n.

Then, m and n intersect in a unique point, say P. 

Thus, through a point $\text{P}$ outside l, there are two lines m and n both parallel to l. This is a contradiction to the parallel axiom.

So, our assumption is wrong. Hence m || n. 

Betweeness - A point C is said to lie between two points A and B, if 

1 A, B and C are collinear points and, 

2 AC + CB = AB.. 

Opposite Rays - Two rays AB and AC are said to be opposite rays if they are collinear and point A is the only common point of the two rays.